Example 27.1. Snowy campus walk.
Dudley stands at the southeast corner of a 1 km\(^2\) square field on campus that is currently covered in snow. He is hurrying to get to his calculus course taking place in the mathematics building at the northwest corner of the field. Dudley plans on first walking (at constant speed) along some portion of the ploughed east-west path that runs along the southern border of the field, and then cutting across the snowy field to head directly toward the mathematics building (at constant speed). Because of the snowy conditions, Dudley can move twice as fast on the ploughed path than he can when walking across the field. If Dudley wants to get to the mathematics building as quickly as possible, how far along the ploughed path should he walk before cutting across the field?
Solution.
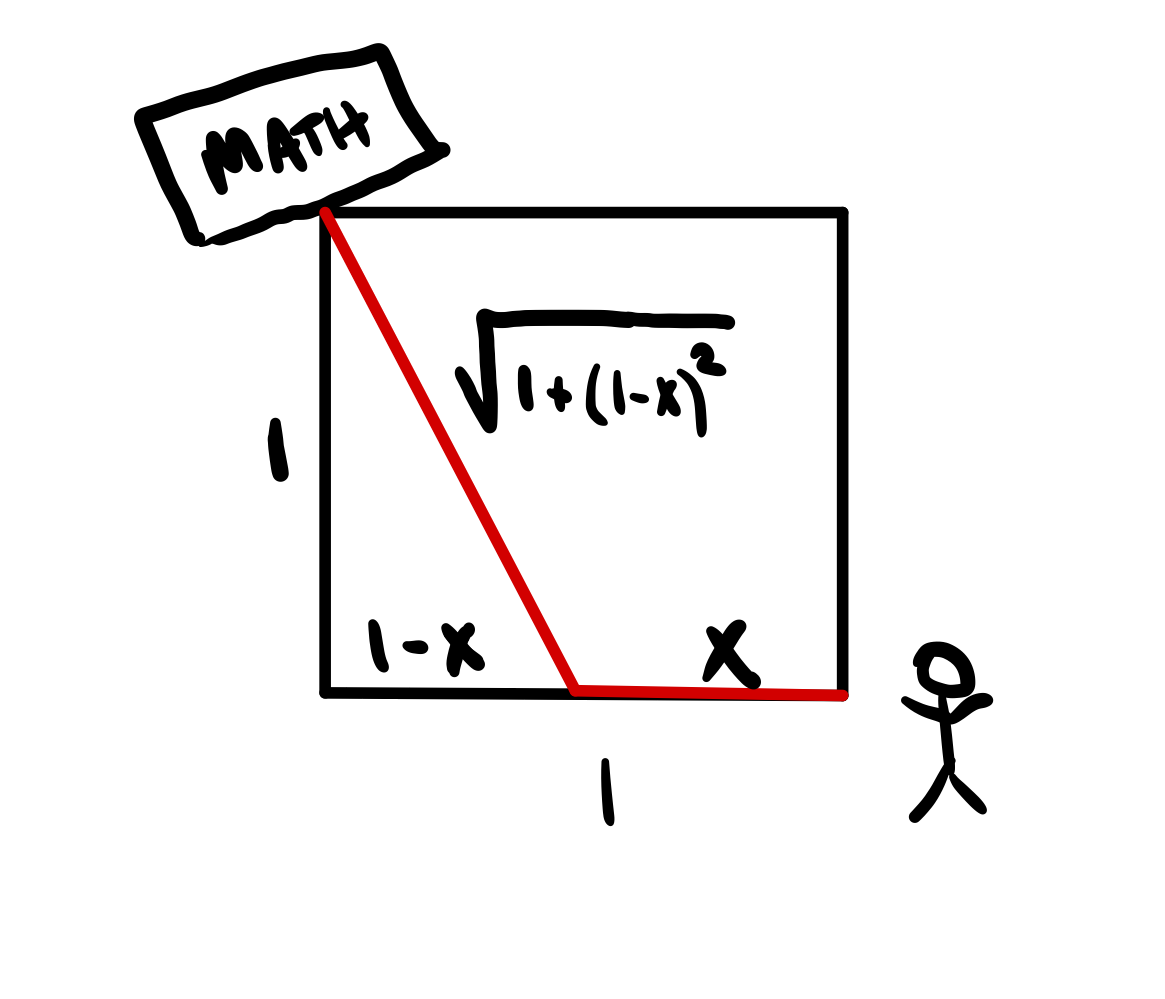
Let \(x\) be the distance Dudley walks along the east-west ploughed path, in which he walks a distance of \(\sqrt{1+(1-x)^2}\) through the snowy field. Let \(s\) be the constant speed at which Dudley can walk through the snowy field; it follows that he moves at maximum speed \(2s\) along the ploughed path. Using the (distance)=(rate)\(\times\)(time) formula for objects traveling at constant speed, we see that the total time \(T\) it takes for Dudley to get to the math building taking such a path is
\begin{equation*}
T=f(x)=\frac{x}{2s}+\frac{\sqrt{1+(1-x)^2}}{s}\text{.}
\end{equation*}
We wish to find the minimum value of \(f\) on the interval \([0,1]\text{.}\) We follow Procedure 20.13. Since \(f\) is differentiable everywhere, its critical points are the solutions to \(f'(x)=0\text{.}\) We solve (treating \(s\) as a constant):
\begin{align*}
f'(x) \amp =0\\
\frac{1}{2s}\amp +\frac{-2(1-x)}{2s\sqrt{1+(1-x)^2}} = 0 \\
\frac{1}{2s} \amp= \frac{1-x}{s\sqrt{1+(1-x)^2}} \\
2(1-x) \amp =\sqrt{1+(1-x)^2}\\
4(1-x)^2\amp =1+(1-x)^2 \\
3(1-x)^2 \amp= 1 \\
(1-x)^2 \amp = \frac{1}{3}\\
(1-x) \amp = \pm \frac{1}{\sqrt{3}} \\
x \amp = 1 \pm \frac{1}{\sqrt{3}} \text{.}
\end{align*}
Thus the only critical point of \(f\) in \([0,1]\) is \(1-\frac{1}{\sqrt{3}}\text{.}\) After evaluating \(f\) at the critical point and the endpoints and doing some careful algebra, we see that
\begin{align*}
f(0) \amp = \frac{\sqrt{2}}{s}=\\
f(1-1/\sqrt{3}) \amp = \frac{1}{s}\left(\frac{1+\sqrt{3}}{2}\right)\\
f(1) \amp = \frac{3}{2s}\text{.}
\end{align*}
Although not totally obvious, you can show by hand that
\begin{equation*}
\frac{1+\sqrt{3}}{2} < \sqrt{2} < \frac{3}{2}
\end{equation*}
from whence it follows that \(f(1-1/\sqrt{3})\) is the minimal value of \(T=f(x)\) on \([0,1]\text{.}\) Thus Dudley should travel a distance of \(1-1/\sqrt{3}\) km along the ploughed path before heading across the snowy field. Godspeed, Dudley!
